Table of contents
Problem - Leetcode
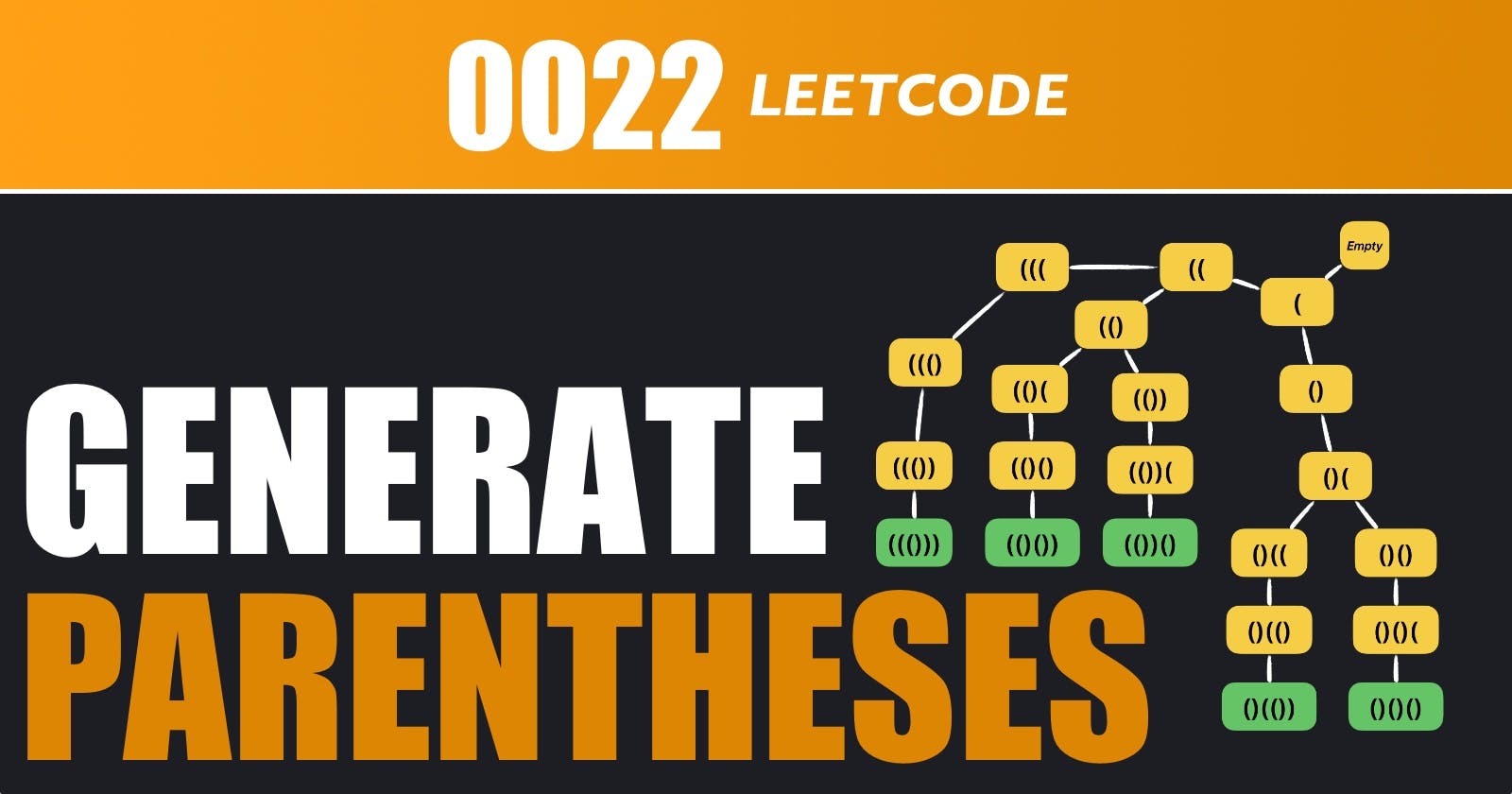
Given n pairs of parentheses, write a function to generate all combinations of well-formed parentheses.
Example 1:
Input: n = 3
Output: ["((()))","(()())","(())()","()(())","()()()"]
Example 2:
Input: n = 1
Output: ["()"]
Constraints:
1 <= n <= 8
Solution in Golang
import "strings"
func generateParenthesis(n int) []string {
var stack []string
var res []string
var backtrack func(int, int)
backtrack = func(openN int, closedN int){
if openN == n && closedN == n && openN == closedN{
res = append(res, strings.Join(stack, ""))
return
}
if openN < n{
stack = append(stack, "(")
backtrack(openN+1, closedN)
pop(&stack)
}
if closedN < openN{
stack = append(stack, ")")
backtrack(openN, closedN+1)
pop(&stack)
}
}
backtrack(0,0)
return res
}
func pop(list *[]string){
length := len(*list)
*list = (*list)[:length-1]
}
This Go code defines a function generateParenthesis that generates all valid combinations of parentheses pairs with a given number n. Here's a detailed explanation of the code:
import "strings": This line imports the "strings" package, which is used for manipulating strings, particularly to join them together.func generateParenthesis(n int) []string { ... }: This is the main function that takes an integernas input and returns a slice of strings representing valid combinations of parentheses.Inside this function, three main variables are defined:
stack []string: A slice of strings used to keep track of the currently open and unclosed parentheses.res []string: A slice of strings to store the final result, i.e., valid combinations of parentheses.backtrack func(int, int): This is a nested function that performs the recursive backtracking to generate the combinations.
backtrackis a recursive function that takes two arguments:openN: The count of open parentheses.closedN: The count of closed parentheses.
The first
ifcondition checks if we have reached the desired count of open and closed parentheses, both equal ton. If so, it means we have formed a valid combination, and it's added to theresslice by joining thestackusingstrings.Join.Next, there are two
ifconditions:if openN < n { ... }: This checks if the count of open parentheses is less thann. If true, it appends an open parenthesis "(" to thestack, increments the count of open parentheses, and then callsbacktrackrecursively. After the recursive call, it "pops" the last element from thestackto backtrack and explore other possibilities.if closedN < openN { ... }: This checks if the count of closed parentheses is less than the count of open parentheses. If true, it appends a closed parenthesis ")" to thestack, increments the count of closed parentheses, and then callsbacktrackrecursively. It also pops the last element from thestackafterward for backtracking.
Finally, the
backtrackfunction is initially called withopenNandclosedNboth set to 0, starting the recursive process to generate valid combinations.The function returns the
resslice, which contains all the valid combinations of parentheses.func pop(list *[]string) { ... }: This function is used to remove the last element from a slice of strings. It's a utility function called withinbacktrackto backtrack and explore other possibilities by removing the last element added to thestack.
In summary, this code uses a recursive backtracking approach to generate all valid combinations of parentheses pairs with a given count n. It maintains a stack to keep track of the currently open and unclosed parentheses and appends and pops elements from it while exploring possibilities. The valid combinations are stored in the res slice and returned as the final result.